Testing > By Test > Tensile Test
Tinius Olsen
Tensile Test

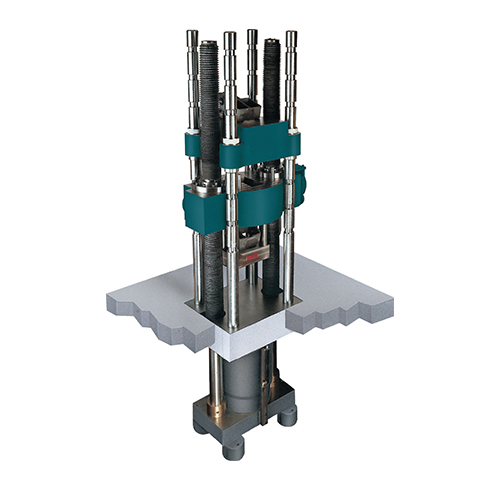
During a tensile test, a clamped specimen is subjected to a constant rate of tensile loading such that it stretches and ultimately breaks. Typically these tests are performed in accordance with an internationally recognised standard procedure and calculation of results so that the data is portable between labs. The rate of loading can be applied as either a constant rate of extension, constant rate of strain or constant rate of load, depending on the standard.
Tensile strength σUTS, or SU, is the stress at which a material breaks or permanently deforms. Tensile strength is an intensive property and, consequently, does not depend on the size of the test specimen. However, it is dependent on the preparation of the specimen and the temperature of the test environment and material.
Tensile strength, along with elastic modulus and corrosion resistance, is an important parameter of engineering materials that are used in structures and mechanical devices. It is specified for materials such as alloys, composite materials, ceramics, plastics and wood.
There are various definitions of tensile strength and the following are representative of the properties for low-carbon steel:
1. Ultimate strength
2. Yield strength
3. Rupture
4. Strain hardening region
5. Necking region
Metals, including steel, have a linear stress-strain relationship up to the limit of proportionality, which is most often close to the yield point. In some steels the stress falls after the yield point; this is due to the interaction of carbon atoms and dislocations in the stressed steel. Work-hardened and alloy steels do not show this effect and for most metals yield point is not sharply defined.
If loading is removed prior to reaching the limit of proportionality, or yield point, the material will return to its initial shape. However, once the yield point is exceeded, the deformation is permanent and the material will not return to its initial shape. This permanent deformation is known as plastic deformation, which is unacceptable for many applications, and so the material’s properties at its yield point are used as the design limitation.
In a normal tensile loading test, steel and many other ductile metals will undergo a period of strain hardening, once the yield point has been passed, in which the stress increases again with increasing strain up to the ultimate strength. If the material is unloaded at this point, the stress-strain curve will be parallel to that portion of the curve between the origin and the yield point. If it is re-loaded it will follow the unloading curve up again to the ultimate strength, which has become the new yield strength.
After a metal has been loaded to its yield strength it begins to “neck” as the cross-sectional area of the specimen decreases due to plastic flow. When necking becomes substantial, it may cause a reversal of the engineering stress-strain curve, where decreasing stress correlates to increasing strain because of geometric effects. This is because the engineering stress and engineering strain are calculated assuming the original cross-sectional area before necking.
If a graph is plotted in terms of true stress and true strain, the curve will always slope upwards and never reverse, as true stress is corrected for the decrease in cross-sectional area. Necking is not observed for materials loaded in compression. The peak stress on the engineering stress-strain curve is known as the ultimate strength. After a period of necking, the material will rupture and the stored elastic energy is released as noise and heat. The stress on the material at the time of rupture is known as the tensile strength.
Ductile metals do not have a well-defined yield point, so in these cases we have to “invent” one. The yield strength is typically defined by the “0.2% offset strain”. The yield strength at 0.2% offset is determined by finding the intersection of the stress-strain curve with a line parallel to the initial slope of the curve and which intercepts the abscissa at 0.2%.
Brittle materials such as concrete and carbon fiber do not have a yield point and do not strain-harden, which means that the ultimate strength and breaking strength are the same.
Typical brittle materials do not show any plastic deformation but fail while the deformation is elastic. One of the characteristics of a brittle failure is that the two broken parts can be reassembled to produce the same shape as the original component. A typical stress strain curve for a brittle material will be linear. Testing of several identical specimens will result in different failure stresses.
Tensile strength is measured in units of force per unit area. In the SI system, the units are Newtons per square meter (N/m²) or pascals (Pa). The Imperial, or English, units are pounds-force per square inch (lbf/in²) or PSI. However, engineers in the USA usually use units of ksi, which is a thousand psi.
Request a Proposal